Integrate sin^2x cos^2x
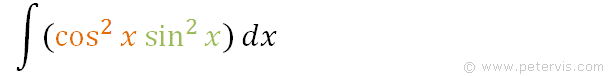
To integrate sin^2x cos^2x, also written as ∫cos2x sin2x dx, sin squared x cos squared x, sin^2(x) cos^2(x), and (sin x)^2 (cos x)^2, we start by using standard trig identities to to change the form.
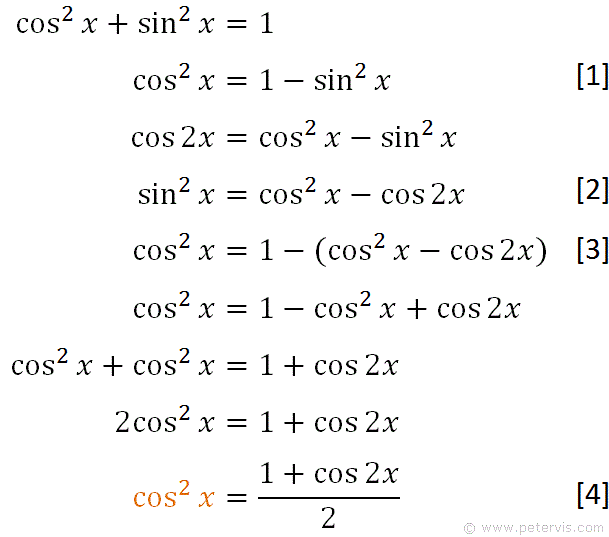
We start by using the Pythagorean trig identity and rearrange it for cos squared x to make expression [1]. We recall the double angle trig identity and rearrange it for sin squared x to make expression [2]. We then substitute expression [2] into expression [1] to make expression [4], which is for cos squared x.
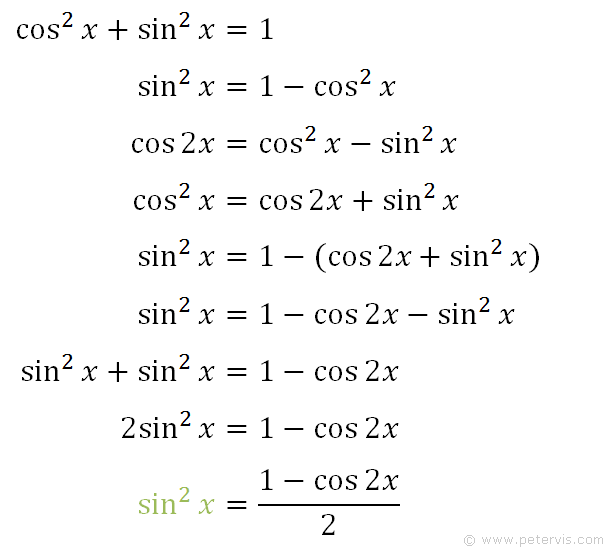
We repeat the previous steps using the Pythagorean and double angle trig identities, except this time we build an expression for sin squared x.
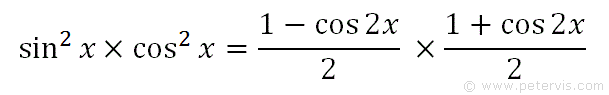
Hence we can rewrite sin^2x cos^2x in a new form that means the same thing.

We focus on multiplying the brackets, and therefore move the fraction out of the way.

We see that the cos2x terms cancel out thereby simplifying the expression.
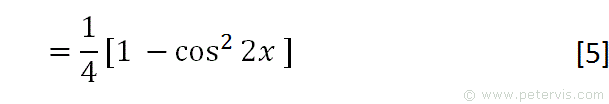
Hence, this is the simplified expression. Sometimes in mathematics, you crawl your way out of a hole, only to fall into another. We still have a cos squared term that will pose some difficulties.

We recall expression [4], which we made previously, as shown above.

If we multiply the angles by 2 on both sides, then the equation remains balanced, however, it gives us cos squared 2x for substitution.

I prefer to have it written like this. We can now substitute for cos squared 2x in our previous equation [5] as shown below.

At this stage, we substitute and simplify.

We recall our original integration problem, and now we can write it in another form as shown on the RHS.

We can integrate each term separately as shown on the RHS. Although the first term on the RHS, which is a constant, is simple to integrate, the second term will need some more thinking. Hence we focus our attention on integrating the second term as shown below in red.

We focus on integrating cos4x and get the answer, as shown in red. We can now substitute this part of the solution into our original integration problem as shown below.
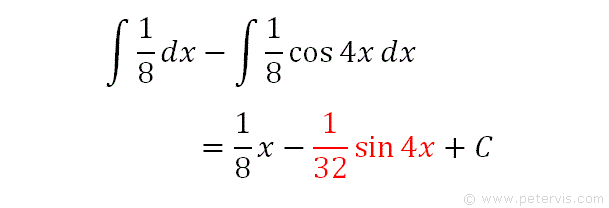
Hence, this is the solution, where C is the integration constant.